About us Sobre nós

Light and Matter

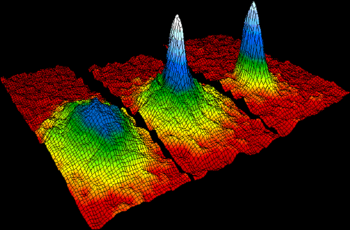
Light enables the test and modification of the properties of matter, and matter can be designed to control, regulate, and manipulate the propagation of light. This bidirectional relation motivated significant research efforts over centuries. In the last few decades, many unconventional geometries of propagation media were realized experimentally, opening the field of topological photonics. Light is also used to engineer virtually any desirable potentials for quantum particles, for cold atoms and their condensates. A large class of systems featuring unusual properties can be identified as regular aperiodic systems, which include almost-periodic media, such as photonic quasicrystals, moiré lattices (for both photonics and cold atoms), and spiral-like potentials. Localization and propagation of light in such media and its interaction with cold atomic gases are the focus of this research stream.